RelBiPermittivity¶
| Type: | 2-Tensor, or section |
|---|---|
| Range: | [v_1, …, v_9] |
| Default: | -/- |
| Appearance: | optional |
This parameter specifies the relative magnetic permittivity (the coupling between the electric displacement 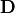 field and the magnetic field
field and the magnetic field  )
) 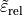 as follows:
as follows:

Warning
Usually the relative magnetic permittivity 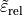 is defined relative to the background permittivity and permeability. We do not follow this convention as it is not clear how this convention is applied for general an-isotropic tensors
is defined relative to the background permittivity and permeability. We do not follow this convention as it is not clear how this convention is applied for general an-isotropic tensors  and
and 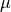 .
.
For general bi-anisotropic materials the material constitutive relations read as
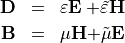
Typically the tensors 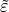 and
and 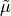 are not independent from each other. To preserve reciprocity of Maxwell’s equation it is required that
are not independent from each other. To preserve reciprocity of Maxwell’s equation it is required that
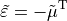
For a bi-anisotropic material without damping additional to the Ohmic losses we have
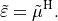
Isotropic materials are typically classified by the reciprocity parameter  and the chirality parameter
and the chirality parameter  ,
,
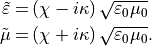
This material definition introduces no additional losses and is reciprocal for 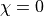 .
.